Lab 19: Magnetic Field and Magnetic Force

 Here, Professor Mason put iron powder into the magnetic bar at random locations, the interesting thing was that the iron powder automatically filled in the direction of the magnetic field, which goes from the north pole to  the south pole.

 Similarly, we used a compass to show the same behavior as the iron powder. We placed the compass on every place surrounding the magnetic bar, and the north direction of the compass always pointed in the direction of the south on the magnetic bar because they attract each other, we signaled it with arrows as we advanced. Also, when we placed the compass at the north pole, it didn't pointed to the south direction, because the repelling force was stronger than the attractive force.

 This is a big magnet. Professor Mason explained that when a magnetic domain is cut in half, then there is no magnetic field, which causes the flux to be zero, and are considered magnetic monopoles, which in reality they don't exist. To prove this, he removed the north pole of the big magnet, the removed piece was not a magnetic monopole because is also has a south pole since all cut poles come in groups, meaning a south and a north.

 We drew surfaces on the magnetic lines. Two of our surfaces had zero flux, which means there is no magnetism. One of the surfaces had 5 magnetic lines going in, which means the magnetic field is negative.

 These were some exercises using the right hand rule.

 Through some unit calculations, we found that the unit for magnetic field is N/A.m

 We calculated the acceleration of the proton to be 3.74x10^11 m/s^2 in the same direction as the force.

 First, we use the force equal the centripetal acceleration. Since we were given a frequency, we use the angular velocity in our calculations. So that, B = mw/q, in order to find the magnetic field of the given problem.

Professor Mason put a string in between the magnet, when he turn the device on, the string was moving up and down because energizing the string causes the electrons to collide.

 We learnt something important, when dealing with protons, we use the right hand rule, and when dealing with electrons, always use the left hand rule.

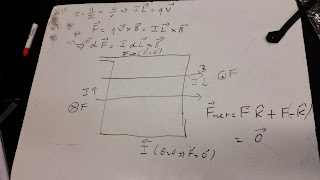
Using the right hand rule with both currents, we found that the net force equals zero.

 The net force in this case is also zero, because the direction of the force is always perpendicular to the displacement of a magnetic field.

We were asked to find the net magnetic forces on a semicircular wire divided into 15 segments with constant magnetic field, radius and current. Excel only calculates radians, for this reason I converted degrees into radians. The net force was calculated with the formula F = IBLsinθ. The force is much more smaller for some segments than for other segments because of the angle it has with respect to the magnetic field. When the angle is nearly perpendicular to the magnetic field, the force is nearly zero, and if the force is parallel to the magnetic force, then it is at its maximum.